Force and Extension
A tensile test involves gripping the dogbone sample at each end and pulling it apart. We then record the pulling force and associated extension of the sample in this destructive test at regular time increments, until the sample fractures.
In English units, force is measured in lb., and the sample’s cross-section–determined by multiplying the actual sheet thickness by the dogbone sample width–in sq. in. Therefore, the English unit for strength is lb./in.2, abbreviated as psi. Most engineered metals have tensile-strength levels in excess of 10,000 psi, leading to a further abbreviation: 1000 psi = 1 ksi. So, 50,000 psi = 50 ksi.
However, most of the world uses the International System of Units (SI), with force measured in N, mass in kg, length in m, and strength in Pa. The tensile strength of most metal alloys exceeds 1 million Pa (or 1 MPa); 1 ksi equals approximately 7 MPa, so 50 ksi = 350 MPa.
Extension represents the increase in length that occurs in a predefined section of the dogbone, known as gauge length. Calculate elongation as the percent increase represented by the extension relative to gauge length. For example, if gauge length before testing is 2 in., and the tensile test results in the distance between the gauge marks increasing to 2.3 in., the 0.3-in. measured extension results in a calculated elongation of 15 percent.
Stress and Strain and Everything Else
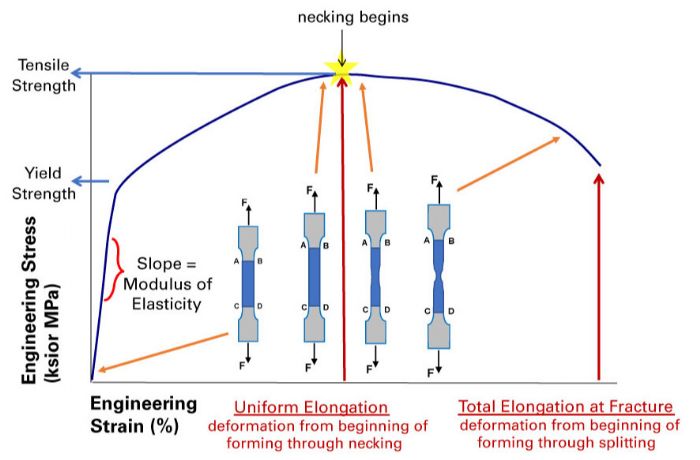
We use force and extension to measure stress and strain, displayed graphically on a stress-strain curve (see the accompanying image). Stress changes as a function of the applied strain.
Application of force leads to deflection of the test sample, and of the sheet metal in a stamping press. Permanent deformation must occur in order for an engineered part to take shape, but no permanent deformation occurs upon removal of a sufficiently low applied force. This is known as elastic deformation; the slope of the stress-strain curve in this linear region represents the modulus of elasticity (or Young’s modulus or the elastic modulus). As the modulus increases, so does a material’s rigidity. Alloys with a higher modulus also exhibit less-severe springback at a given strength.
After applying a critical load, the stress-strain response no longer remains linear. And, after load removal and relief of the elastic strains, some permanent deformation remains. At this point, the material has yielded, with this critical load known as yield strength. High-yield-strength products are more difficult to form in a stamping press, as well as deform in an automobile crash.
Increasing the applied load, either during a tensile test or as the press ram approaches bottom dead center, leads to the sheet metal gaining strength, through a process called strain hardening or work hardening. We use the slope of the stress-strain curve in this region to calculate the strain-hardening exponent (n-value). High-n-value metal grades distribute strains more uniformly in the early stages of load application, delaying strain localization and early failure.
As the dogbone sample elongates during the tensile test, its width and thickness decrease. We use the changes in width and thickness to calculate the plastic strain ratio (r-value), also called the Lankford coefficient or plastic-anisotropy ratio. Higher-r-value alloys possess increased resistance to thinning, critical when deep-drawing cups and cylinders.
If the dogbone sample were able to maintain its original cross-sectional area, then work hardening would require ever-increasing loads to pull the sample apart. In reality, the shrinking width and thickness decrease the cross-section as the test progresses. Since strength is load divided by cross-sectional area, at the beginning of the test the increase in work hardening is more significant than the cross-section decrease, which leads to a positive slope in the stress-strain curve. After a critical amount of deformation, the impact of the cross-section decrease takes over, and the calculated strength appears to decrease, resulting in a negative slope in the stress-strain curve.
Ultimate tensile strength (or tensile strength) is the stress at the apex of the stress-strain curve; the strain at this point is called uniform elongation. The name comes from the rectangular profile within the gauge region that exists from the beginning of the test until reaching uniform elongation. Characterization of the post-uniform elongation zone includes a rapidly shrinking cross-section evidenced by local thinning, called a neck. Strains localizing to form a neck indicate a high stress area and a loss of load-carrying ability. Uniform elongation indicates the beginning of necking, which occurs prior to fracture. A local neck prevents the forming of a deeper part profile and serves as a crack-initiation site. Additional loading, including fatigue loading during the life of the part, may cause a neck to progress to fracture.
Total elongation at fracture represents the strain associated with complete separation of the dogbone test sample. The certified material-properties document included with a coil shipment typically includes this value. Instead, stampers should consider evaluating uniform elongation to better characterize formability. And, taking on additional significance in understanding the failure behavior of advanced high-strength steels: the reduction of area measured at the broken faces after the test sample fractures.
Remember that generating tensile-test results occurs with a test coupon pulled lengthwise, which is free to contract in the perpendicular direction. In contrast, the product shape constrains material flow in engineered stampings. MF
Industry-Related Terms: Alloys,
Center,
Conductivity,
Ductility,
Form,
Forming,
Gauge,
Impact Resistance,
Ram,
Tensile Strength,
Thickness,
Work HardeningView Glossary of Metalforming Terms
See also: Engineering Quality Solutions, Inc., 4M Partners, LLC
Technologies: Materials, Quality Control
 All materials possess physical and mechanical properties. Measurement of physical properties occurs without changing the material itself, and includes density, mass, melting and boiling points, and electrical and thermal conductivity. These remain constant under the same composition, temperature and pressure.
All materials possess physical and mechanical properties. Measurement of physical properties occurs without changing the material itself, and includes density, mass, melting and boiling points, and electrical and thermal conductivity. These remain constant under the same composition, temperature and pressure.







