Understanding the Behavior of Sheetmetal
June 1, 2009Comments
Highly diverse topics have been presented in this column over the past decade. In contrast, for the next half year this column will explore more deeply the fundamentals of how and why sheetmetals deform. The target audience is the personnel in metalforming companies, and especially the workers on the press floor. These personnel are being exposed to new alloys, advanced high-strength steels, new techniques to process sheetmetal into useful parts, special coatings/lubricants, and other sometimes drastic changes that conjure up questions and misunderstandings. Therefore, the explanations will be logical and readily understandable. Simple illustrations will add additional clarification. Some metallurgists may take exception to the simplicity of the explanations. However, all are designed for press-shop utilization.
The series of columns will include:
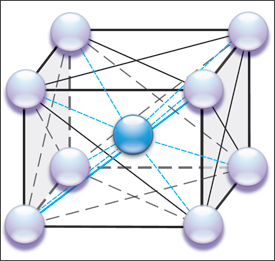 |
| Fig. 1—Schematic shows the multiple atomic force interactions (represented as lines) between the nine atoms (body-centered cube) making up an iron (steel) unit cell. |
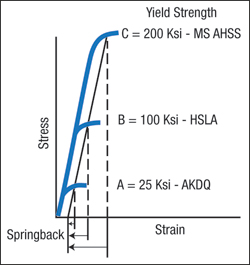
1) Elastic stresses (springback)
2) Yielding (start of permanent deformation)
3) Deformation (work hardening)
4) Failure (necking and fracture)
5) Forming limits (total elongation and forming limit curves) and
6) Surfaces (roughness and coatings). This month covers the fundamentals of elastic stresses and springback.
Understanding springback begins at the atomic level. Elastic stresses hold the atoms in place in the unit cell (Fig. 1), which is iron with a very small amount of carbon. The spheres represent iron atoms. Since the unit cell for iron has the body-centered cubic (BCC) configuration, there are eight corner iron atoms (pearl spheres) and one central iron atom (blue sphere). Each unit cell shares its six faces and corner iron atoms with six adjoining unit cells. This configuration replicates itself until a sheet of steel has length, width and depth.
The lines connecting the spheres in Fig. 1 depict the elastic stresses that bind the atoms together. Instead of lines of elastic stress, now visualize the iron atoms to be connected by tension/com-pression springs. Applying an outside force to the metal causes the spacing of the atoms to increase (tensile force) or decrease (compressive force). This creates a change in the stress between the atoms and an unstable state maintained only by the outside forces. Removal of the outside forces allows the elastic spacing between atoms to return back to their stable positions, causing the metal to springback to its original shape. The springs are still extended or compressed under load, but are back in their initial condition that balances the unit cell to its least energy state. A common concept is that the metal is attempting to return to its original shape. This is true only if no plastic (permanent) deformation or shape has been added that prevents the metal from returning to its initial state. Examples are hemispherical domes, closed end channels, deep-drawn cups, and other shapes that mechanically do not allow the return of the sheetmetal to its initial state. In this case, some elastic stresses cannot return back to their initial stable, lowest energy state. These remaining elastic stresses are called residual or trapped stresses.